This story may seem to have nothing to do with flying airplanes but it does, the lesson at the end definitely pertains to how we should fly jets.
— James Albright
Updated:
2016-02-21
The photo shown here was presented by then 18-year old Robert Harmon to my wife-to-be, who kept hearing stories about Bob, his philosophies, and his wonderful stories about engineering, life at Purdue, and our lives to come as Purdue graduates. Four years later I graduated with a degree in Industrial Engineering which I used quite a bit as an Air Force and then civilian pilot. Bob graduated with a degree in Electrical Engineering and went on to work on all matter of turbine engines which eventually led him into Industrial Engineering. We shared a few classes during our first year and he soundly beat me at our first exam in Statics 101. That's how I learned all about the . . .
The Harmon Rule of Error
Life before calculators
In 1974 the electronic pocket calculator was just coming into being. You could buy one for about $100 and would have something that could add, subtract, multiply, and divide. I built one from a schematic presented by Popular Electronics magazine and was probably the only freshman engineering student at Purdue to have one. But it didn't matter, we were not allowed to use them. Our lives were ruled by slide rules.
A slide rule typically has upper and lower fixed slats, known as "stators," and a sliding slat in the middle known as a "slide." You could multiply, divide, and do much more complicated things by positioning the numbers on the slats relative to each other and searching for the answer opposite another number. There was a problem, however. The numbers appeared in finite ranges, depending on the scales, and it was up to you to figure out where to put the decimal point.
If, for example, you need to multiply 2.3 by 5.7, you moved the slide to place the "1" index on the slide's top scale underneath the third tick mark between 2 and 3 on the lower scale of the upper stator. You then look for the seventh tick mark between the 5 and 6 on the slide. You would see the answer on the bottom scale of the upper stator. Looking at the photo above, you see that ends up being 131, or maybe it is 13.1, or perhaps 1310. Wait, maybe it might be 1.31. So which is it?
With a slide rule, you need to do a little extra work to figure this out. Well if the problem was 2 x 5 the answer would be 10. If it was 3 x 6 it would be 18. So we know the answer is between 10 and 18. That means our answer is 13.1.
Of course that isn't really accurate by today's standards. But the slide rule got us pretty close. As engineers we would note that the 13.1 has a probable error of 0.1 since we couldn't read the scale with any greater precision. If we used this number in subsequent calculations, the errors would compound.
Statics 101
The study of statics concerns the equilibrium of bodies under the action of stationary forces and is one of the first courses most engineers have to master before moving on to loftier concerns. Bob and I sat next to each other in class. Bob eventually became a serious student and mastered everything he needed to know. But that was still in his future. He didn't really pay close attention during that first semester and I expected to beat him soundly on the first exam. I did not.
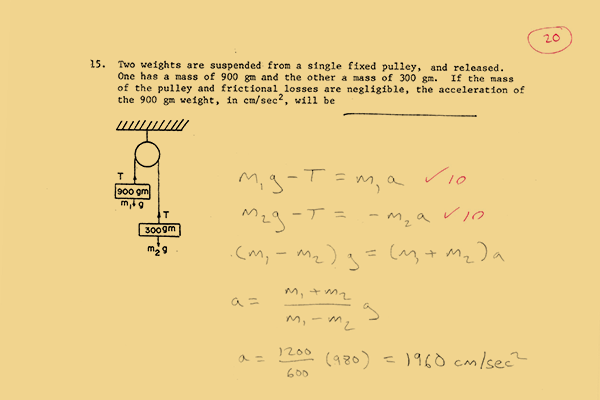
The way these exams worked was you got points for just constructing the basic formulas correctly. In this example I managed to get both the required formulas and earned 10 points for each. Unfortunately, I divided the (m1 - m2) into the (m1 + m2) instead of the other way around and put the a and g where they needed to be and got the wrong answer. So chalk up 20 points for knowing the formulas but mucking up the math.
Bob got the two formulas right for 20 points; but he also got the right answer for a cool 30 points.
I guess it kind of grated on me that he did so much better than me. I asked to see his work so I could learn from them and realized that he lucked into the right answer. The gravitational acceleration, g, is 980 cm/sec2 and must be factored into the equation. But Bob simply took that, divided by two, and called it good. The ratio between the difference in mass of the two weights and their total is also 2. Did Bob do all that in his head and realized the answer was 980 divided by 2? I had to know.
"Bob," I asked, "why did you divide 980 by 2?"
"Well it's like this," he said, signalling another famous Bob story. "The Harmon Rule of Error says that if you are going to make a mistake, it will more than likely be by a factor of 2, 5, or 10."
Of course that is true, but I never thought of it so plainly. If you didn't get the decimal point in the right place with your slide rule, the answer would be off by a factor of 10. But it you went on to use that erroneous number in future calculations, the error could be by a factor of 2 or 5, depending on how you used the number. It was brilliant. Of course it didn't answer my question but it gave me another arrow in my quiver of rules to live by.
"What's all this mean to a pilot?"
We often do simple math in the cockpit or allow the computers in the cockpit to do this math for us. While these electrons are not subject to possible errors from a slide rule they are subject to the Harmon Rule of Error because of input errors. We can input the wrong numbers or previous inputs can corrupt the current inputs.
- Let's say you have been instructed to cross a fix 50 nautical miles ahead of you at an altitude that is 15,000 feet below you. You program the flight management system and it happily tells you there is nothing to do for ten minutes, but fear not, it will let you know when the time comes. So you continue with your sandwich and forget about it. Then, with only 10 miles to go, the nose pushes over, the speed builds to the red line, and the same computer tells you the descent cannot be made without shedding parts from the airplane as it approaches terminal velocity. Waaah?
- In this example we failed to remember or realize there had been an earlier input for a much gentler descent that ended up only 5,000 feet below you at a fix 40 nautical miles ahead. The airplane simply reported the first descent was easy and left the aerobatics after that for a future warning.
- The Harmon Rule of Error simply tells us that we need to apply that slide rule common sense check to everything. Just as we needed to do a rough approximation to place the decimal point in our slide rule math problem, we need to do the same when ensuring the flight management system is behaving. We should have realized losing 15,000 feet in 50 nautical miles is a "now" action, not a "later" action. More about how to figure your descents: Descent.