Amaze your friends, stun your enemies! That was the purpose of learning this technique. I can't see a need for it today, but I can't give it up either . . .
— James Albright
Updated:
2013-11-23
Our once trusty EC-135’s were falling apart on us. Most of the civilian world had already abandoned the Boeing 707 as too costly to operate and too unreliable to use. We were spending more and more time baby sitting broken aircraft on the road and it was getting old. It was time to leave; I was nearing my fifth year with the operation and had progressed from copilot, to pilot, to instructor pilot, and finally to evaluator pilot. “Those who can’t fly teach,” the saying went. “Those who can’t teach evaluate,” I added.
In truth, I wanted to instruct. But even more, I wanted to explore more ways to make math work in the cockpit. I had a year with nothing to do but give check rides, fly missions, and ponder the arithmetic with the objective of conquering all “wags,” death to TLAR: “That Looks About Right.”
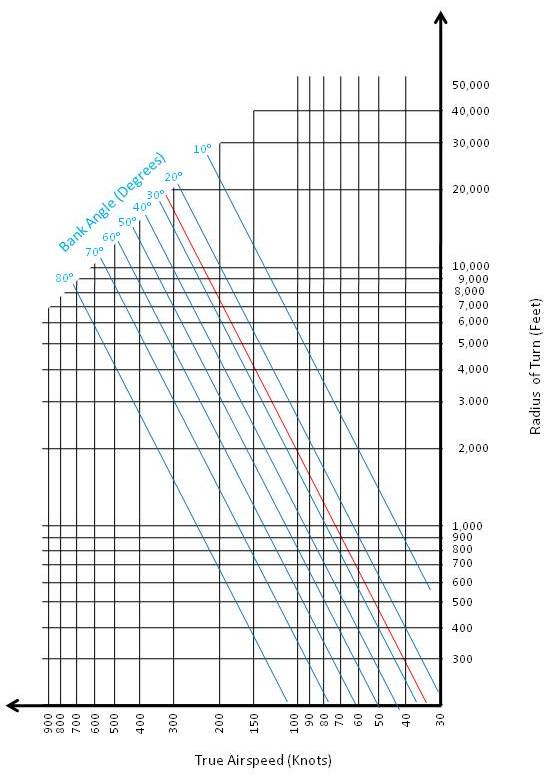
At the Air Force Instrument Instructor’s School, the fundamental calculation was 60-to-1 for all things vertical and horizontal. The vertical was proven beyond a shadow of a doubt. The horizontal? Not so much. The starting point for horizontal math was turn radius, which was taught as:
Unless it was:
But these often didn’t work. Let's say you were maneuvering for the procedure turn at 180 knots, or 3 nautical miles per minute. Using the first formula you had a turn radius of 1 nautical mile, using the second it was 0.9 nautical miles. Pretty close. But what about at 120 knots, or 2 nautical miles per minute? The answers were 0 and 0.4 nautical miles. Nonsense! It was obvious the first formula was wrong, but what of the second?
Many of the calculations were based on 30 degrees of bank, but most modern aircraft with flight directors used less bank. Some of the calculations were based on an early aircraft instrument called the turn indicator which provided for a standard rate turn of 2 minutes for a 360 degree turn. While that worked in Wilbur and Orville’s day, at the speed many jets fly today that would take even more than 30 degrees of bank. With the Boeing 707 a standard rate turn was usually too steep, so the flight director defaulted to 25 degrees of bank. Calling around to other units, it seemed 25 degrees was the magic number.
Finding a formula for turn radius an engineer would be happy with wasn’t a problem:
Plugging in the tangent of 25 degrees, the conversion from nautical miles per hour (S) to nautical miles per min (V), and the conversion from feet to nautical miles yielded something suspiciously like one of the school rules of thumb:
Solving...
The difference between 8.9 and 10 in the denominator is insignificant. Checking a range of bank angles shows the error some have noted with the original rule was due to variations in bank angles. At 25 degrees of bank, the rule appears to be accurate within ten percent. As we said in instrument instructor’s school: “close enough.”

At 25 degrees of bank, turn radius is equal to nautical miles per minute squared, divided by ten.
Does the rule have anything at all to do with sixty-to-one? No. Does the rule make flying easier with math? Yes. The rule is a keeper.
The rule also illustrated that some of the classic TLAR wags were pretty good. Perhaps a role of math was to validate the good ones and throw out the bad. The classic TLAR was the holding pattern teardrop. I had come to use what my children would call “guess and check” for the holding patterns in the local area around Hawaii. We flew them so often that I could make note of what worked and what didn’t.
Instrument Instructor’s School came to the rescue with another formula of unknown origin:
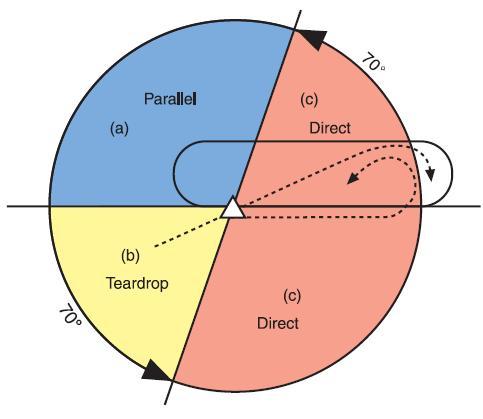
When entering a holding pattern, your turn direction and heading are usually very well defined, based on your entry sector. If, for example, you entered within 70 degrees of the inbound course, you turned outbound in the direction of holding. It was cut and dried. If, however, you were “conveniently aligned,” you could fly a heading of your choosing. Ideally this heading would place you with enough offset so that your inbound turned rolled you on course. But what was that offset? It was, more times than not, a wag.
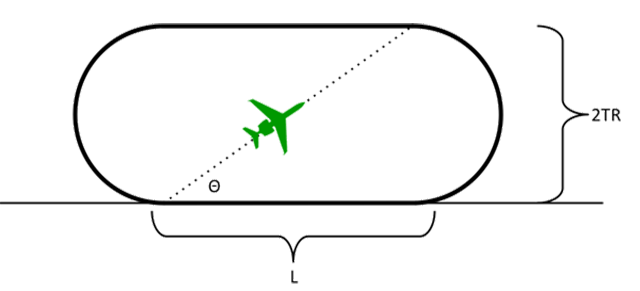
Finding an exact formula was problematic, in that the holding pattern teardrop is not symmetrical, the distance of the teardrop leg is slightly longer than the inbound leg. But the arctangent was pretty close.
In most parts of the world, holding pattern speed is limited to around 200 knots, certainly not more than 250 knots. The range of speed would be bound by 2 nautical miles per minute on the low side up to no more than 4 nautical miles per hour on the high. That placed turn radius between .4 and 1.6 nautical miles. Leg length would be between 5 and 10 nautical miles.
A check of a few examples:
| nm/min | (nm/min)2/10 | leg | arctan(2r/leg) | 120r/leg | error |
| 2 | 0.4 | 5 | 9 | 10 | 11% |
| 10 | 5 | 5 | 0% | ||
| 3 | 0.9 | 5 | 20 | 22 | 10% |
| 10 | 10 | 11 | 10% | ||
| 4 | 1.4 | 5 | 29 | 34 | 17% |
| 10 | 16 | 17 | 6% |
Conclusion? Close enough!

A holding pattern teardrop angle of 120 times the turn radius divided by leg length will provide an on course roll out, no wind.
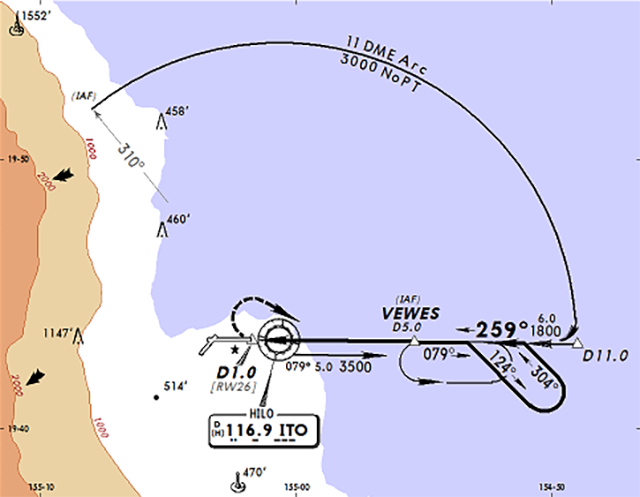
My work in the Hawaii instrument pattern was almost done, but there was one more dragon to slay. An approach into Hilo, Hawaii had become an obsession because most our guys were bracketing the arc. That is, they were flying a series of straight legs to keep the bearing pointer more or less on a wing tip, and flying more or less on course. Of course this is what most pilots did and even a recommended technique in most instrument manuals. I experimented over the years and came up with a bank angle to fly a smooth, on course, arc. But that was after much trial and error. I wanted an answer obtainable the first time, with math.
The instrument school answer was to use a bank angle equal to the turn radius times 30 divided by the arc distance. I tried it the first chance I got and it worked. I started flying most of these procedures at 180 knots to make the math easy: 3 nautical miles per minute. That made the turn radius 1 nautical mile and the bank angle for the Hilo arc came to a meager 3 degrees. It worked.
Solving for the bank angle and converting to nautical miles per minute yielded:
In our example V = 180 and the turn radius is 11 miles:
The error of the rule of thumb was less than 20 percent and who can read 0.53 degrees on an attitude indicator? The school house solution was a winner.

To fly an arc around a point, use a bank angle equal to the turn radius times 30 divided by the arc length.
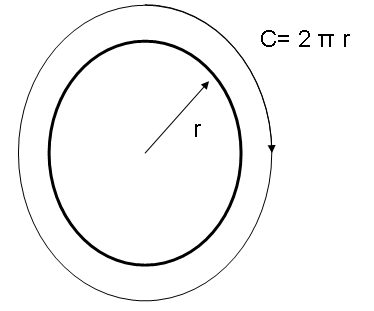
As long as I was on the subject of arcs, another school rule of thumb had been bugging me. They taught the distance along an arc is equal to the arc radius times the number of degrees divided by 60, another sixty-to-one miracle. Of course it had nothing to do with the sixty-to-one relation, but the method did work. Why? An arc is a segment of a circle, and the distance around a circle is also known as its circumference. Everyone knows that formula:
That would be the distance around all 360 degrees. If you wanted the distance of just one degree:
And you can get the distance of any arc by multiplying that by the number of degrees:
Using 3.14 for π and simplifying the equation:
While it wasn’t sixty-to-one, it was pretty close.

The distance traveled along an arc is equal to the arc radius times the arc angle divided by 60.
Thirty years later, I still use these formulas now and then. But more often than not, the electrons are doing the thinking for me.