I will admit that I am a recovering engineer turned pilot who still enjoys a good differential equation or two. But I am recovered enough to acknowledge you don't need anything more than basic math to fly an airplane. But if you want to really understand your craft, you need to know something about aerodynamics. And all that begins with the purest form of physics: the field of mechanics. This most recent update is meant to complement the free PDF you will have received in a newsletter. You are subscribed, aren't you?
— James Albright
Updated:
2025-03-01
So before we begin our coverage of aerodynamics, a quick primer on mechanics is in order. The United States Air Force requires its safety officers to pass this course and it is of course a prerequisite for test pilot school. Mechanics makes a better pilot. So why not give it a try?
Note: we aviators do things in nautical miles because the math works better for navigation (1 degree of latitude = 60 nautical miles). So the math that follows is based on 1 nautical mile = 6,076 feet. The math on this page will work in statute miles (1 statue mile = 5,280 feet) but the answers will necessarily be different.)
1
Motion
In the study of physics, we often consider fictitious objects that have no mass or friction to eliminate inacuracies and just get the formulas to work. That helps us to master the concepts before we tackle the real life problems. Let's not do that. Let's ignore friction and inacuracies and just talk about getting from Point A to Point B. That's what we do as pilots. And motorcyclists.
Position
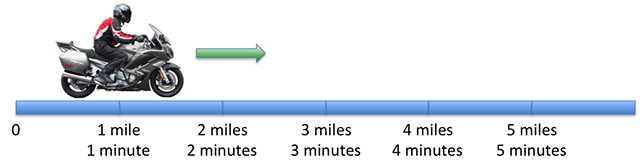
First things first: we need to discuss basic position in space. Starting with two dimensions, we can measure our position forward with positive numbers, backwards with negative numbers. In the drawing, the bike is at 0.
Speed (Instantaneous)
If after 1 minute the bike has traveled 1 mile, we can compute the speed thusly:
Or, preferring a more standard measure:
Speed (Average)
Of course the speed you see on the speedometer isn't necessarily the speed you will accomplish over the duration of the trip. To figure average speed, you need a reference point at the start and at the end of the time in question. An odometer makes as good a reference as any, say you start the trip with the odometer reading 001000 miles.
If after an hour the odometer reads 001060, your average speed has been:
But let's say you run into traffic and after two hours the odometer reads 001090, your average speed has fallen to:
Velocity
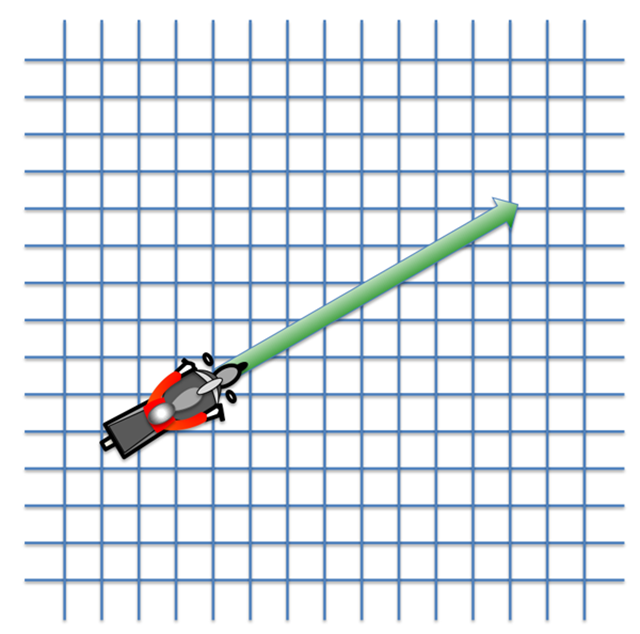
So far we've discussed speed, which is a scalar quantity. That is, it has a magnitude but no direction. As pilots, we are interested in velocity, which is a vector quantity. It has magnitude and direction.
You can think of direction as angles on a grid, or more conveniently, angles on a compass. We can say in our drawing the motorcyclist is heading east-north-east, 30° north of east, or best of all, heading 060.
Where speed is simply a number, to the physicist velocity is an arrow. The length of the arrow shows magnitude, the direction shows, well, direction. Sure, to a pilot velocity is a number. But always keep in mind there is a direction associated with that number.
From all this we can derive a basic equation for velocity:
Through the magic of algebra:
and:
Consistent Units
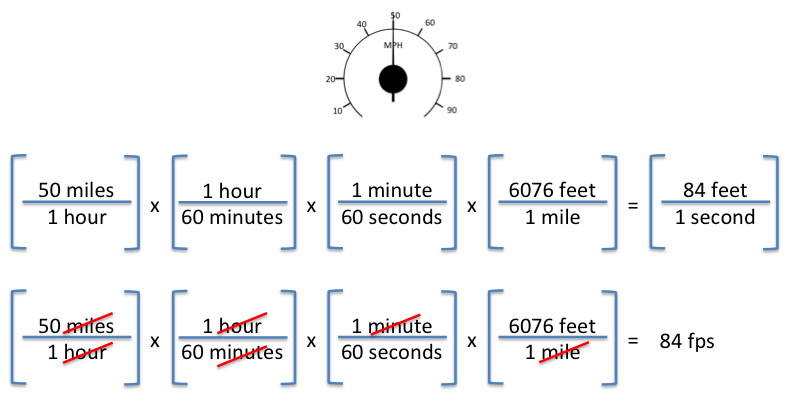
Before we go any further, let's talk about units. Miles versus feet, hours versus seconds, it can be a real mess. It doesn't matter what you use, but when the units you choose must be consistent in every equation. So for the sake of example let's say you are going 50 mph, miles per hour. To convert that into feet per second you need only multiply it by 1 set to units of your choosing. 1 hour divided by 60 minutes, for example, is equal to one. You can multiply your starting number with these conversion factors, just so long as all the units are multiplied through as well. In the example, the hours, minutes, and miles all "cancel" out to reveal the answer: 84 feet per second.
Velocity on a Graph
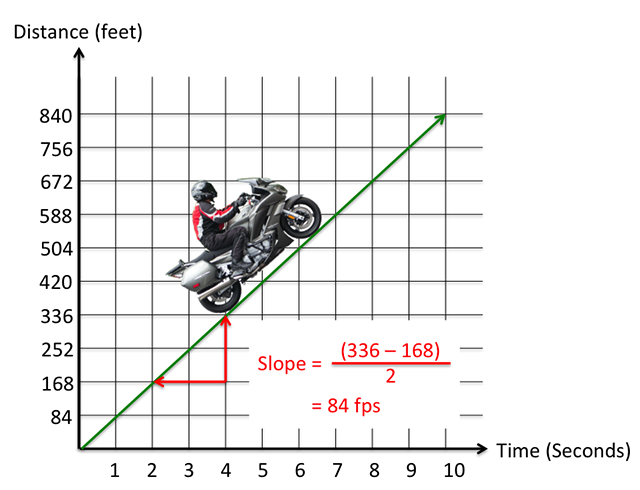
You can also plot distance versus time to come up with a graphical representation of velocity. The slope of the distance change along any point of the line will show the velocity over that time span.
Since our velocity is constant — neither accelerating or decelerating — any two points will yield a velocity of 84 fps.
Acceleration
We define acceleration as the change in velocity per unit of time. If, for example, we go from 0 mph to 50 mph in 10 seconds, we have a mathematical mess on our hands. Converting the velocity into feet per second, as we saw above, makes things more sensible.
The "feet per second square" is a standard unit of acceleration
If we establish the symbol Δ, delta, as a change in values, Δv denotes the change in velocity. Ergo:
Acceleration on a Graph
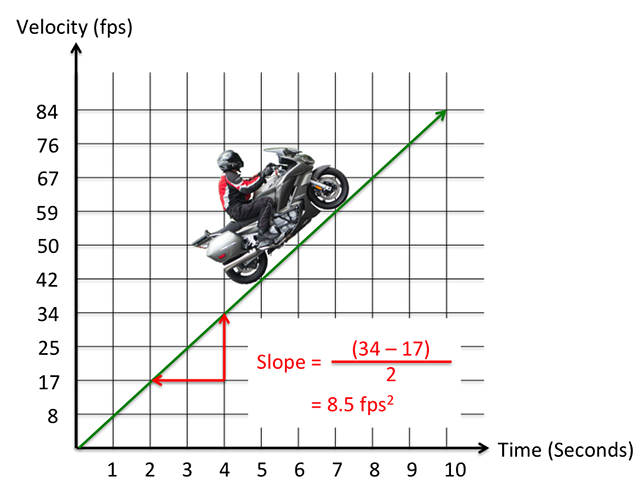
Just as you can also plot distance versus time to come up with a graphical representation of velocity, so to can you plot velocity versus time to come up with a graphical representation of acceleration. The slope of the velocity change along any point of the line will show the acceleration over that time span. Since our acceleration is constant any two points will yield an acceleration of 8.5 fps2.
If a = v / t, and we know that it is, we can do some algebra and find that v = at. Using that, we find that the average velocity of something accelerating at a constant rate a is (0 + at) / 2. Well, zero is an easily eliminated term isn't it?
But we already know d = vt, so that means d= (½at) t, so:
Jerk
Jerk is a subject for sophomore physics to provoke sophomoric laughter from the back of the class, but it is a legitimate measure of mechanics that is especially pertinent to pilots, be they jerks or not. How does the motorcycle rider experience jerk? Perhaps we should go back to the beginning.
Sitting on the bike with the motor off and two feet planted on the ground, the rider has a velocity of zero. Because there is no change in velocity, acceleration is also zero.
Cruising along at a steady 50 mph, the velocity can be said to be 84 fps. Because the speed is constant, the acceleration is again zero.
If the rider spots a squirrel on the road and decides to brake, the velocity drops off. If the rider is smooth on the brakes, the acceleration might be constant. Let's say the rider goes from 84 fps to a full stop in 30 seconds. The acceleration is:
Note that we say the acceleration is negative in this instance, it is a deceleration.
If the acceleration is constant, then jerk = 0. Let's say the rider goes from moderate braking, around -2 fps2, to harder braking, around 5 fps2, in the last 5 seconds:
You won't find a jerk meter on the bike (or in your cockpit), but you will know it when you feel it. (Jerk is usually accompanied by irregular head bobbing.)
Jerk on a Graph
Now let's say our intrepid rider underestimates the amount of braking required to avoid the rodent. If he or she then decides to really clamp down on the brakes, then backs off, and then on again, the stop will be said to be "jerky." Or in my case, when I do this with the lovely Mrs. on back, I will have been said to be a jerk.
Why is this especially pertinent to a pilot? There are lots of reasons covered in the story Smoothness, but here's a sample. You can stop the airplane on a short runway using aggressive braking by applying your best guess of brake pedal and then holding the brakes steady. As the brakes heat up they become more effective and the deceleration will increase. But it will be done smoothly. Or you can apply some brake, a little less, a little more. If the Mrs. is on board, you will be the jerk pilot up front.
Falling
Though it isn't something we pilots like to consider, the field of mechanics is concerned with the act of falling. Galilei Galileo, 1564 - 1642, was able to hypothesize that absent air resistance, all objects fall with the same acceleration regardless of mass. He did this with the clever use of ramps, but tests in vacuum chambers confirm that he was right.
Over the years we've come to realize that objects on earth fall at 32 ft/sec2 which is 9.8 m/sec2 for most of the world. When speaking of acceleration to earth — falling —, we substitute the term g for a so that:
and:
2
More math
Mass
Since Galileo went through all that trouble to invent gravity for us, we should apply this knowledge to our discussion of mass . . .
Mass is a measure of the amount of material in a body. Weight, on the other hand, is a force caused by the gravitational attraction of the earth, moon, sun, or other heavenly bodies. Weight will vary, depending upon where the body is located in space. Mass will not vary with position.
Source: Dole, pg. 2
Rearranging:
This mass unit is called the slug.
Moments
Moments are measured by multiplying the amount of the applied force F by the moment arm l.
Source: Dole, pg. 5
The moment arm is the perpendicular distance from the line of action of the applied force to the center of rotation. Moments are measured as foot pounds (ft-lbs) or as inch pounds (in.-lb). If a mechanic uses a 10 in. long wrench and applied 25 lb of force, the torque on the nut is 250 in.-lb.
The aircraft moments that are of particular interest to pilots include: pitching moments, yawing moments, and rolling moments. Some of these moments may oppose each others in direction. It is therefore necessary to classify each moment, not only by its magnitude, but also by its direction of rotation. In the case of pitching moments, a nose-up or nose-down classification seems appropriate. Mathematically, it is desirable that moments be classified as positive (+) or negative (-). For example, if a clockwise moment is considered to be a + moment, then a counterclockwise moment is considered to be a - moment. By definition, aircraft nose-up pitching moments are considered to be + moments.
Equilibrium Conditions
There are two requirements for a body to meet to be in a state of equilibrium:
- There must be no unbalanced forces acting on the body. This is written as the mathematical formula Σ F = 0. Σ (cap sigma) is the Greek symbol for "sum of."
- There must be no unbalanced moments acting on the body. Mathematically, Σ M = 0.
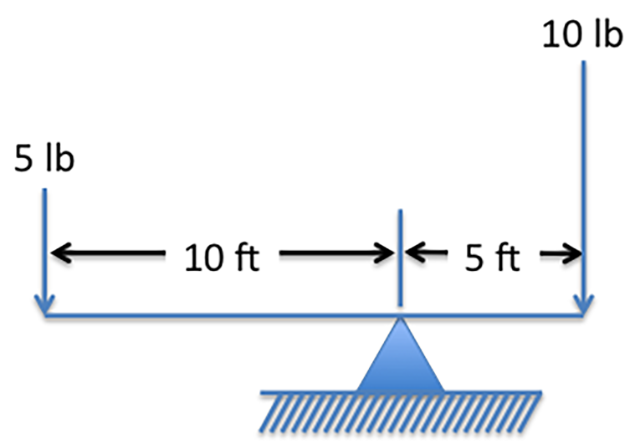
Moments at the fulcrum in [the figure] are 50 ft-lb clockwise and 50 ft-lb counterclockwise. So Σ M = 0. To satisfy the first condition of equilibrium, the fulcrum must press against the seesaw with a force of 15 lb. So, Σ F = 0.
Source: Dole, pg. 6
3
Newton's laws
If you really want to understand . . .
Newton's First Law
"A body at rest will remain at rest and a body in motion will remain in motion, unless acted upon by an unbalanced force."
The first law implies that bodies have a property called inertia. Inertia may be defined as the property of a body that results in its maintaining its velocity unchanged unless it interacts with an unbalanced force. The measure of inertia is what is technically known as mass.
There are several points to be made about this first law that will make the laws that follow easier to, well, follow:
- When there are no external forces, objects move with constant velocity.
- Inertia is resistance to changes in motion.
- The amount of inertia an object has is measured by its mass; more mass means more inertia.
Newton's Second Law
"If a body is acted upon by an unbalanced force, the body will accelerate in the direction of the force and the acceleration will be directly proportional to the force and inversely proportional to the mass of the body."
Acceleration is the change in motion (speed) of a body in a unit of time.
The amount of acceleration a is directly proportional to the unbalanced force F and is inversely proportional to the mass m of the body. These two effects can be expressed by the simple equation:
or more commonly:
Don't forget that g is a for objects on earth falling to earth. That is weight, isn't it? Yes it is:
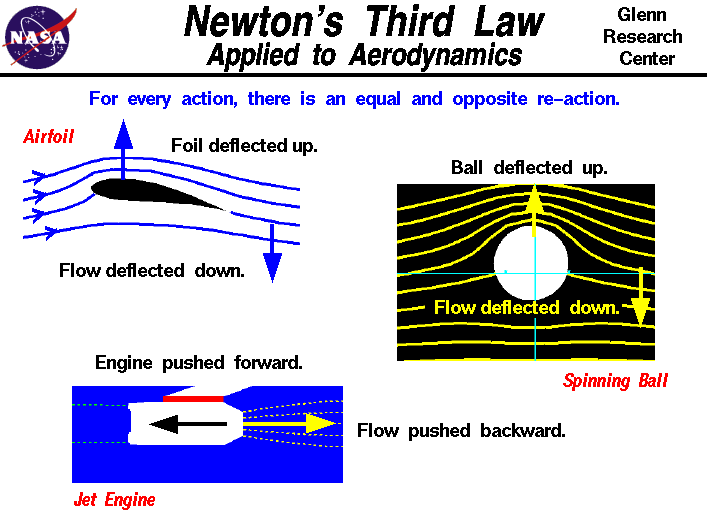
Newton's Third Law
"For every action force there is an equal and opposite reaction force."
- The motion of lift from an airfoil, the air is deflected downward by the airfoil’s action, and in reaction, the wing is pushed upward.
- The motion of a spinning ball, the air is deflected to one side, and the ball reacts by moving in the opposite
- The motion of a jet engine produces thrust and hot exhaust gases flow out the back of the engine, and a thrusting force is produced in the opposite direction.
4
More formulas
Work
In physics, work has a meaning different from the popular definition. You can push against a solid wall until you are exhausted but, unless the wall moves, you are not doing any work. Work requires that a force must move an object in the direction of the force. Another way of saying this is: "Only the component of the force in the direction of movement does any work."
Source: Dole, pg. 8
Energy
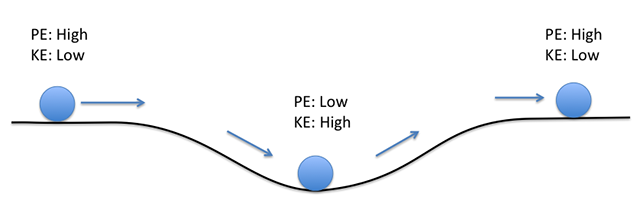
Consider for a moment a metal ball traveling at a constant speed on a flat surface approaching a dip, as shown in the drawing. As we did in freshman engineering class, we say the ball and the surface are both frictionless and there is no air resistance for the sake of this academic exercise.
We know that as the ball goes down the dip in the surface, its speed will increase until it gets to the bottom where it will trade that extra speed to climb the ramp. When it ends up back at the original level, since we are discounting the effect of friction, it will again be at its original speed.
Before it hits the slope, the energy of the ball can be described as the Kinetic Energy (KE) that comes from its motion and the Potential Energy (PE) due to its higher elevation.
Energy is the ability to do work. There are many kinds of energy: solar, chemical, heat, nuclear, and others. The type of energy that is of interest to us in aviation is mechanical energy. There are two kinds of mechanical energy. The first is called potential energy of position, or more simply, potential energy (PE). No movement is involved in calculating PE. A good example of this kind of energy is water stored behind a dam. If released, the water would be able to do work, such as running a generator. PE equals the weight W of an object multiplied by the height h of the object above some base plane:
The second kind of mechanical energy is called kinetic energy (KE). As the name implies, kinetic energy requires movement of an object. It is a function of the mass m of the object and its velocity V:
The total mechanical energy TE of an object is the sum of its PE and KE:
The law of conservation of energy states that the total energy remains constant. Both potential and kinetic energy can change in value, but the total energy must remain the same; "Energy cannot be created or destroyed, but can change in form."
Source: Dole, pg. 8
Power
Power is defined as "the rate of doing work":
Source: Dole, pg. 9
But distance / time = velocity, so:
James Watt defined the term horsepower as 550 ft-lb/sec, therefore:
If the velocity is measured in knots rather than feet per second, the formula becomes:
Friction
There are two basic types of friction: static and kinetic. If you try to push an object across a table, at first it resists with great force until it breaks free, and then it takes less force to continue pushing it. Static friction exists when the objects are stationary and varies up until the point it gives in. Kinetic friction occurs when the objects are moving and is less than static friction.
If two forces are in contact with each other, then a force develops between them when an attempt is made to move them relative to each other. This force is called friction. Several factors are involved in determining friction effects on aircraft during takeoff and landing operations. Among these are runway surface material, condition of the runway, tire material and tread, the amount of brake slippage, and others. All of these variables determine a coefficient of friction μ (mu). The actual braking force Fb is the product of this coefficient μ and the normal (squeezing) force between the tires and the runway:
Source: Dole, pg. 10
where Fb = braking force
μ = coefficient of friction
N = normal force on wheels.
One of the perks of hosting this website is that I get all kinds of mail from all over the world and, in the end, it all adds up to my reservoir of knowledge. I've never considered myself a natural pilot but more a natural engineer turned pilot. Here is a letter with the engineering gift from a very young age.
Dear Sir,
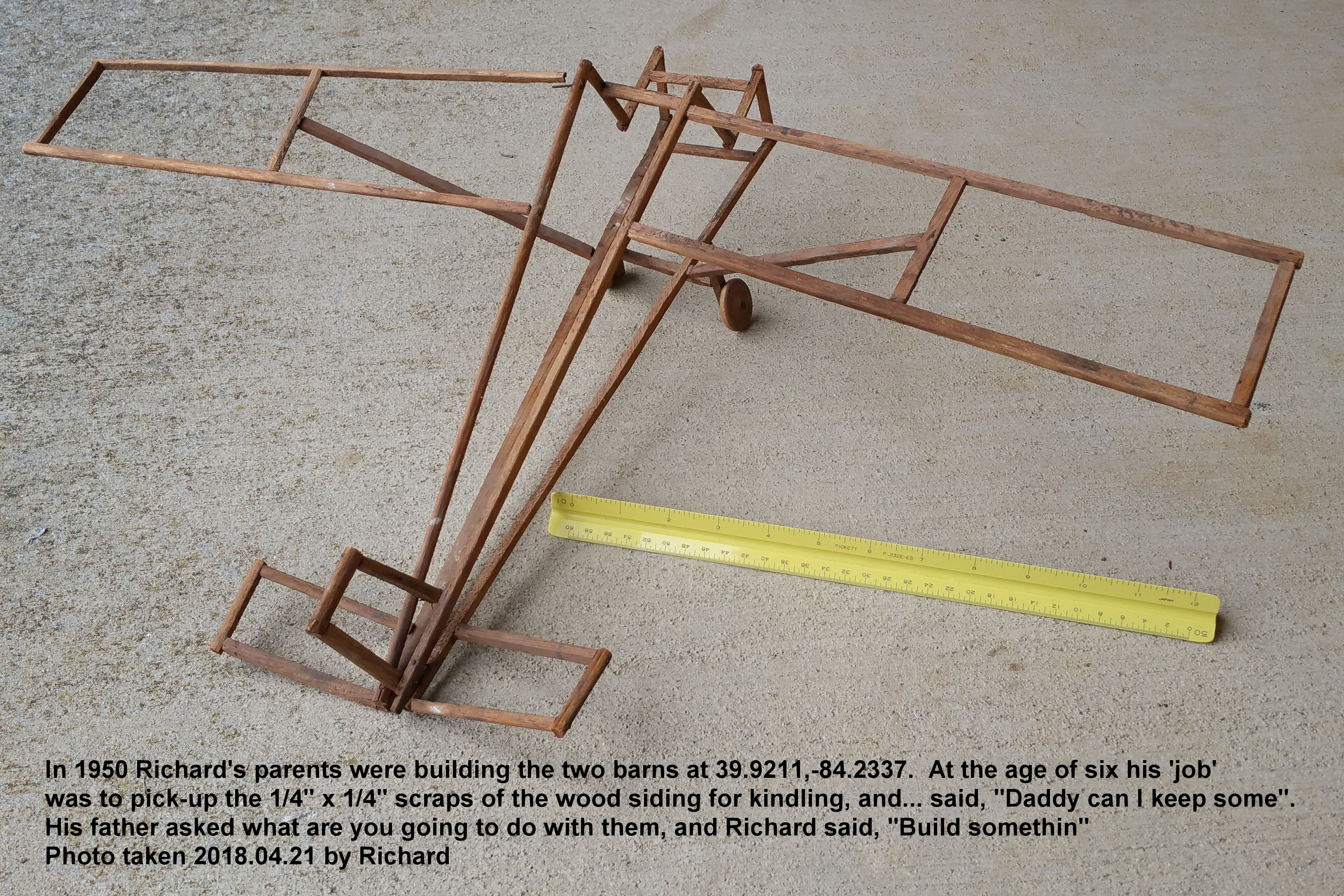
I grew up on a working farm in west central Ohio. In 1950 my father was building two barns (they are still is good shape just north of KDAY) and at the age of six my job was to pick up the 1/4" x 1/4" trimmings of the tongue and groove siding for use as kindling. I asked my father if I could have some and he asked what I was going to do with them. I simply answered, "Build somethin".
A few days later I used a hack-saw (nice fine teeth) to cut the wood pieces to matching lengths and then with VERY small brad finishing nails, I nailed together thus creating a 'stick' frame ~24" wingspan high-wing 'tail-dragger' airplane. I still have it. I looked at it recently and recognized the thought process required to fabricate and assemble this simple 'structure'.
Circa 1956 Piper came out with the TriPacer. I sent and requested information. I still have the envelop and brochure. It contained, what I now know as a Top-Front-Side picture with ~3" wing span. I used a 6" steel thin ruler graduated in 1/64" and enlarged the three views to ~20" wing span. Then, fabricated ribs and bulkheads and finally using 1/64 sheet balsa wood created a scale replica. I used straight-pins to fabricate hinges for the doors AND movable control surfaces. I did not know that the rudder was controlled by foot pedals. When I opened the two doors and with a finger on each hand turned the 'steering-wheel' to the Left the rudder moved 'correctly'. And, pushed forward on the 'steering-wheel' the elevators dropped for the correct pitch action. I did not realize that there were two control surfaces on the main wing. So I missed the 'mark' for roll-control. I was sorta half-right with the flaps as I called them 'air-brakes' and moved them with a lever INSIDE the cockpit. Yes, I still have ALL the above including the envelope.
When a senior in high school I made a Smoke Tunnel and also a Wind Tunnel. Plotted L/D curves for various airfoils and photographed the stream-lines over the same at various AOA. Missed a Superior rating at the State of Ohio Science Fair at Toledo by one point....
In Fall of 1962 I was about to enter the 'new' College of Aerospace Engineering at the University of Cincinnati. During my evaluation of the several Engineering Disciplines, something called Civil Engineering was an order of magnitude higher on my self made evaluation scoring scale. I was very good at it. At the conclusion of the five year Bachelor program, they made me a deal by giving me money (I was the Teaching Assistant and 'ran' the hydraulic lab) AND paying my tuition to get my Masters.
I full-filled all the requirements and shortly thereafter became a Registered Professional Engineer. You can check that at the State of Ohio Registration web site.
My father's next younger brother started flying in the mid 40's. He will be 94 this August, just passed his Class III medical, is current and flies his Piper 180HP Challenger out of L36.
In late 1970's I acquired my Private Pilot License. My sons's mother did not like flying so that took a sabbatical. About 2003 I re-took ground school so as to catch-up with anything new and started to fly again. I have VERY low hours and looking to start up ....again.
I stumbled across your web site and book by doing an Internet search for "airplane turn radius as function of velocity". I have some suggestions for the fellows at iFly GPS. I REALLY liked the Vista-Nav system, owned and used it. Was paying my subscription to Honeywell until they stopped supporting it around 2012.
Regards,
Richard Oaks
References
(Source material)
Dole, Charles E., Flight Theory and Aerodynamics, 1981, John Wiley & Sons, Inc, New York, NY, 1981.